HANDBOOK OF THE INTERNATIONAL COMPARISON PROGRAMME

PROCESSING OF THE BASIC DATA

1. Purchasing-power parities for basic headings
1. The EKS method
2. The CPD approach
2. Aggregation of the basic heading parities up to the level of GDP
1. The G-K and EKS methods in brief
2. Linking of regional results and the fixity question
3. Extrapolation of benchmark estimates to other years

1. PROCESSING OF THE BASIC DATA

210. This chapter briefly sketches the methods of processing the price and expenditure data provided by countries to the ICP coordinators. Section A takes up the calculation of parities at the basic heading level. Ube discussion in this section is fairly technical because it is felt that a good understanding of estimation of the detailed heading parities should make it possible for country statistical offices to better appreciate the type of price information required by the ICP. Considerable space is therefore given to the basic heading parities with the view that this will help improve the overall quality of the comparisons. Section B concerns aggregation from the basic heading level to GDP. Many of the technical aspects of the methods are discussed in Annex II. Section C of this chapter briefly discusses the question of extrapolating benchmark estimates to years other than the benchmark reference year, a task that country statistical offices may be called upon to perform.
211. By way of contrast, less space is devoted to aggregations above the basic heading level. This is partly because these index number problems have been the subject of a number of expert group meetings over the past several years, and so there is ample material available discussing the issues. 16/ Further, there is no unanimity about how the aggregations should be made to build up either regional or world comparisons, so the Handbook will simply sketch some of the issues and methods employed. One general question is whether there should be symmetry in the methods employed to obtain parities at the basic heading level and those used to aggregate the basic headings. This issue was discussed in the expert group meetings, but no consensus was reached; in the Handbook, the methods of obtaining basic heading parities are treated independently from aggregations of the basic headings.

212. The parity at the basic heading level is an average of the individual item price ratios of the specifications belonging to a given basic heading. This section discusses two principal approaches to estimating these basic heading parities, namely, the Èltetö-Köves-Szulc (EKS) and the country-product-dummy (CPD) methods. The principal differences in the estimates generated by these two methods arise at the level of the basic heading; as one moves to aggregations of the basic headings, the overall results are unlikely to be affected by which method is chosen. Both methods are described here because both have been extensively used in ICP work.
213. For purposes of illustration of how parities are obtained for basic headings, a price tableau for a basic heading for four countries for eight specifications will be used. In this illustration, no item weights are provided but the countries have been able to indicate whether items are important in their consumption by an asterisk (*). In this example, country A will be taken as the numeraire, and price ratios between all pairs of countries are given in rows (5) to (10), with those with respect to country A given first. The six price ratios given will be termed direct price ratios because they are formed directly by taking the prices of the two countries, as in (B/A). An indirect price ratio derived from two direct ratios, as the product of [(B/A) x (C/B)), will be denoted (C/A)^.

Tableau of Prices and Price Ratios

	Items
	1	2	3	4	5	6	7	8
Countries	Prices
(1) A	2*	6*	--	--	10	--	1*	4
(2) B	12	35	3*	5	40*	--	--	18
(3) C	25	50	7	12*	--	10*	--	--
(4) D	150*	400*	--	100	--	70*	80	--
Country/Country	Price ratios
(5) B/A	6	5.83	--	--	4	--	--	4.50
(6) C/A	12.5	8.33	--	--	--	--	--	--
(7) D/A	75	66.67	--	--	--	--	8.0	--
(8) C/B	2.083	1.429	2.333	2.40	--	--	--	--
(9) D/B	12.5	11.429	--	20.0	--	--	--	--
(10) D/C	6.0	8.0	--	8.333	--	7.0	--	--

214. Binary comparisons at the basic heading level are quite straightforward. Consider countries A and B in the example above. The parity between A and B for the category is taken as the geometric mean of the price ratios for the matching items 1, 2, 5, and 8, which, in the example, is 5.01 = (6 x 5.83 x 4 x 4.5 )^1/4 . As noted, no item weights are given. However, where the asterisked item system is used, importance of items is taken into account in the following way: whenever items are marked with an asterisk in one of the two countries, they are included in the calculation of the parities. In the example above, only items 1, 2 and 5 would be included in the comparison of A and B because these items have an asterisk (*) in at least one of the two countries. The parity between A and B estimated on the basis of asterisked items would be 5.19 = (6 x 5.83 x 4)^1/3.
215. The price tableau in the illustration has a number of items for which countries have not provided prices, which is the usual situation. Suppose, however, that we consider a complete tableau of only items (1) and (2), where each country has provided prices for both items. In this case, the binary comparisons between each pair of countries is transitive so that (C/A)^ = (C/A), that is, the direct parity between C/A would be equal to the product of the parities B/A and C/B. This can be seen below, where the price ratios are repeated for items (1) and (2) from the price tableau above, and the geometric mean of the price comparison is given for all possible binary comparisons:

	B/A	C/A	D/A	C/B	D/B	D/C
Item 1	6.00	12.50	75.00	2.083	12.50	6.00
Item 2	5.833	8.333	66.67	1.428	11.43	8.00
Geometric mean	5.916	10.206	70.71	1.725	11.95	6.93

The geometric mean of B/A is equal to (C/A)/(C/B), that is, 5.916 = 10.206/1.725, and so forth for any other direct and indirect binary comparisons. Also, use of geometric averages produces end results that remain base country invariant, i.e. they are not influenced by which country played the role of numerator and which of denominator. (With arithmetic averages, this would not be the case, since the unweighted arithmetic average of the A/B ratios is not the reciprocal of the unweighted arithmetic average of the B/A ratios.)

216. In chapter III a desirable property of the geometric mean was mentioned in connection with time-to-time indexes. The property is that the ratio of the geometric mean of two series is equal to the geometric mean of the product of the ratios of the two series. In the above example, using only items 1 and 2, we may note that the geometric mean of prices in B (12 x 35)^1/2'divided by A (2 x 6)^1/2 is 5.916 = 20.494/3.464. This leads us to a discussion of the Èltetö-Köves-Szulc (EKS) method, which allows estimation of transitive multilateral parities based on all possible binary comparisons.

217. When the price tableau is complete, we have noted that the direct binary parity between B and A is equal to the indirect binary derived through third countries such as C or D. However, this is not the case when the price tableau is incomplete, as can be seen from the geometric mean given below based on the full price tableau:

	B/A	C/A	D/A	C/B	D/B	D/C
Geometric mean	5.01	10.206	73.681	2.021	12.132	7.274
Geometric mean*	5.19	10.206	73.681	2.366	11.953	7.274

The first row above gives the geometric mean of the price ratios between each possible pair of countries, using all the prices in the price tableau, while the second row only uses price ratios where the item has an asterisk (*) in at least one of the countries. Considering (B/A), the direct ratio, it can be seen that it does not equal the indirect ratio, (B/A)" = (C/A)/(C/B) in either row. 17/ Or, put another way, transitivity is lost.

218. The EKS method permits transitivity to be restored by taking into account the indirect and direct comparisons by the formula in the following equation:

(1)


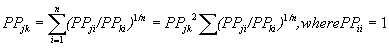
The term "PP" is used to denote a parity at the basic heading level. In EKS, the direct parities (PP_ji, where i = j) and (PP_ki, where i = k), are each counted, while each indirect parity is counted once. In the above example with four countries, the EKS calculation of the (C/A) parity from the geometric mean for the asterisk (*) approach is:

C/A = [ (C/A) x (C/A) x ( (C/D) x (D/A) ) x { (C/B) x (B/A) }] ¼, or

C/A = (10.206 x 10.206 x 10.130 x 12.280]1/4 = 10.670.

219. All the EKS estimates, using all prices, and the asterisk approach are given below:

	B/A	C/A	D/A	C/B	D/B	D/C
EKS	5.267	10.167	70.352	1.930	13.357	6.920
EKS*	5.173	10.670	70.710	2.063	13.669	6.627

220. An advantage of the EKS method is that it produces transitivity and makes use of all the price information available, including both direct price comparisons between each pair of countries, and all indirect price relationships between each pair of countries and the remaining countries. The EKS method is derived from a minimization procedure that was basically mathematical in formulation, though it can also be derived in a weighted form from some general considerations of consumer behaviour. The CPD method that follows is derived from an explicit model of how the price tableau is generated. 18/

221. An alternative way of dealing with an incomplete matrix of prices is the country-product-dummy (CPD) procedure developed by Robert Summers (see Summers, 1973). It has been employed in the ICP calculations for the initial studies, although in recent years most regions have preferred to adopt the EKS method. CPD is a multilateral method in which regression analysis is used to obtain transitive parities for each basic heading. The prices are regressed against two sets of dummy-variables: one set contains a dummy for each specification and the second set a dummy for each country other than the numeraire country. The transitive parities are derived from the coefficients of the country dummies. The estimating equation is as follows:

(2) ln P_j/k = b₁X₁ + b₂X₂ + . . . + b_n-1X_n-1 + Z₁Y₁ + Z₂Y₂ + . . . + Z_mY_m + u,

where n = number of countries, m = number of items in a basic heading, j = 1,2,...,n-1; k = 1,2 .... m, and where ln P is the natural logarithm of the price of an item k in country j. Each of the n-1 countries being compared, other than the numeraire country, is represented by an X dummy variable, and each of the m items in the heading is represented by a Y dummy variable. The country coefficients, the bs, are the natural logarithm of the estimated country parity for the heading, and the item coefficients, the zs, are the natural logarithms of the estimates of the item prices in the currency of the numeraire country.

222. The CPD estimates given below are based on the price tableau, using all the observations in paragraph 213. The very high correlation is spurious since it basically results from explaining the variance in the initial observations owing to different currency units. Similarly, the size of the t statistics on the item coefficients is of only limited application. However, the country and item coefficients are of interest, particularly in column (3), where they are given in their exponentiated form. The coefficients for each country are pps in terms of currency unit of the country compared to the numeraire country A, and the item prices are the estimated average price of each item expressed in the currency unit of country A.

CPD regression example

Variable	Coefficient	t statistic	PPP and item price estimates
Country B	1.574	17.55	4.83
Country C	2.315	22.44	10.12
Country D	4.296	43.88	73.44
Item 1	0.805	8.77	2.24
Item 2	1.766	19.24	5.85
Item 3	-0.422	-3.26	0.66
Item 4	0.171	1.51	1.19
Item 5	2.208	20.60	9.10
Item 6	-0.030	-0.23	0.97
Item 7	0.043	0.39	1.04
Item 8	1.351	12.60	3.86
adj R2 = 0.998 n = 21 df = 11 where n number of price observations and df the degrees of freedom

223. Turning first to the country estimates, the value of country A is 1.0, since it is the numeraire. If these coefficients are compared to the EKS estimates in paragraph 219, the largest difference is for B, about 9 per cent. As was mentioned earlier, if there are no holes in the price matrix, then the CPD and EKS estimates are identical and all direct and indirect binary parities between countries are transitive. The more prices missing from a given price matrix, the less reliable are either the EKS or the CPD estimates compared to a direct country-to-country comparison using the geometric average of price ratios; and the more prices missing, the larger will be the differences between the EKS and the CPD estimates. It is not possible to say which method is closer to the truth; both are approximations.
224. The CPD method estimates a common item price in the currency unit of the numeraire country that, together with the heading parity, in effect produces a full price matrix. The item prices given above are a part of the estimation procedure of the CPD that are of considerable interest in themselves since they are an estimate of the average price for each specification in the currency of the numeraire country across the group of countries. In regional comparisons, for example, a by-product of application of the CPD method in the ESCAP region in 1985 was a set of Asian item prices. These CPD prices provide a basis of comparison for any country in a region of their specification prices with the average. They also have applications where it may be desired to link a country that did not participate in the benchmark comparison to an existing ICP study. For example, for a country whose prices were available at a later date than the initial CPD for a region, it is possible to link the country to a regional or world comparison in the following way: first, the item prices within a basic heading for such a country would be divided by the CPD estimates of the same item prices in a numeraire currency; then the geometric mean of these price ratios would be calculated to provide the basic heading parity that would allow the country to be linked to the regional and world comparison. This exercise was carried out as a non-official research exercise for Taiwan Province of China, based on the CPD average ESCAP prices for 1985.
225. The EKS and CPD systems can also be used with weights for the individual items, or by use of the asterisk (*) system. In phase IV, for example, the CPD system was used for 20 core countries that were used to link the various world regions. Some core countries had items marked with an asterisk (*) and these were given larger weight than non-asterisk items within any basic heading.
226. The Handbook considers the CPD and the EKS as two alternative methods for the multilateral parity calculations at the basic heading level, without trying to give a clear preference to one or the other. The actual conditions in a given region as well as the preferences of regional experts and organizers should determine which of the two methods is applied.

227. Once parities are obtained for each basic heading the aggregated results have to satisfy the basic requirements of international comparisons, commensurably. Expenditure data must be converted by these parities from national currency to the currency of the numeraire country or to an international currency unit. When expenditure data are converted by parities into a common currency and unit of account at the basic heading level, they are then comparable across countries. Thus, by dividing the expenditure of country A by the expenditure of country B in the same currency, interspatial quantity indexes can be obtained for each basic heading. At the basic heading level the quantity estimates vary in reliability and the volume of data is large so they usually are not published. However, the basic heading data are the building blocks necessary to obtain the converted aggregates for both the summary categories and GDP.

228. To aggregate the basic heading parities and expenditures, a method adopted from the suggestion of Geary has usually been employed in comparisons at the regional as well as the world level. 19/ This method is known as the Geary-Khamis or G-K method and produces transitive comparisons between all countries. The EKS and other methods proposed for aggregation also produce transitive results at the level of GDP.
229. A principal advantage of the G-K method is that it produces additive results that have the property of matrix consistency, where the results can be compared down the basic headings and across countries for any basic heading or aggregation. There are strong arguments that gross domestic product should retain this property even after conversion to another currency since it is in accord with standard national accounting practice. Such additive consistency is advantageous not only because it permits an easier analysis of the structure of the aggregates (e.g., it enables the calculation of distribution percentages), but also because it allows comparison across countries.
230. Not all index formulas provide additive results. Neither the Fisher ideal formula (the geometric mean of the Laspeyres and Paasche formulas), nor any method based on the Fisher formula (such as the EKS) will meet the additivity requirement. Nor do chain indexes, where different weights are used in the different defined composite elements (in the various bilateral comparisons), meet this requirement.
231. Any method of aggregation uses some implicit or explicit set of weights for the importance of each country in the comparison. In the usual G-K application, countries are accorded the weight of their own total GDP in the aggregation. 20/ This accords with standard national accounts methodology, where prices embedded in national accounts are an average weighted by the quantities produced in each region.
232. Most other methods of aggregation use a weighting system that accords the same importance to each country. For example, the implicit weighting system of EKS type systems gives the same importance to, say, Luxembourg, as to France, even though France's economy is over 50 times larger than that of Luxembourg. As a consequence, the different aggregation methods produce different results at the aggregate level. These questions are discussed in more detail in annex II, but as an empirical generalization it can be said that systems such as EKS tend to produce somewhat larger differences between per capita incomes between rich and poor countries than the G-K method. All of the aggregation systems proposed produce results much closer to one another than to the nominal results obtained by converting by exchange rates.
233. While aggregation methods such as the EKS and G-K systems move us towards better measures or real output between countries, there is not yet agreement on criteria that would allow one to say that one system was to be always used. To summarize, the main claim for the G-K method is that it follows the conventions of national income accounting and produces additive results. As discussed in annex II, the EKS system may have more basis in consumer theory than the G-K. In G-K, the quantity of each item of a country is the weight, while in EKS each country is given equal weight. There remain differences among the experts on which system should be adopted, and in phase VI it is likely that the results of both methods will be presented. 21/

234. There is one more problem that arises when passing from the regional comparisons to the world comparison. If the G-K or another aggregation system is applied at the regional level, results will be expressed at regional average prices, which vary from region to region. How should the results of the world comparison be expressed? If world average prices are used as weights, the results obtained in the world comparison between any two countries belonging to the same region may be different from those obtained originally in the regional comparison.
235. Many users and producers of ICP results would like to avoid having two different relationships between, say, France and Italy, depending on whether the result was obtained from an EC comparison or a world comparison where different international prices prevail. This view is especially strong in those regions where ICP results are also used for administrative purposes, as in the European Community. It explains why, in phases IV and V, the ICP organizers accepted the so-called "fixity principle", which requires that results obtained in a regional comparison remain unchanged in a comparison covering a larger number of countries.
236. The price to be paid for complying with the fixity requirement is relatively high. Essentially the matrix consistency of the G-K method must be given up at the world level if the fixity principle is applied. 22/ However, this limitation is only observed in official publications. For purposes of research concerned with the structure of the world economy across regions, individual researchers or research organizations may aggregate the basic heading data in other ways that may be more suitable for the analysis of the economic structure of countries.

237. Typically, benchmark estimates are obtained every five years. However, since benchmark estimates are not available until at least two or three years after the benchmark year, this means that the latest available benchmark estimate for a participating country may be from two to eight years prior to the current year. This is one reason why countries often need to approximate estimates between benchmarks. In the case of the OECD countries, these extrapolations are regularly published with estimates of real GDP and the implicit PPPs moved backwards and forwards from the latest benchmark estimate. The European Community has gone further in this direction, moving towards annual benchmarks. For EC, this partly reflects the fact that operational uses of real output numbers often require very current estimates. 23/
238. The general method of extrapolation is quite straightforward. OECD, for example, can take a benchmark GDP estimate of each country for, say, 1985 and extrapolate it forward and backwards by the national growth rate of GDP for each country. The benchmark estimate is in 1985 dollars and the entire series for non-benchmark years will also be in 1985 dollars. One can easily obtain an implicit purchasing power parity from this type of extrapolation. 24/ This discussion has been framed in terms of national growth rates as the basis for extrapolation and it should be noted that one could also have extrapolated PPP for GDP using the implicit deflator.
239. The same method can be used for any sub-aggregate of GDP for which national growth rates (implicit deflators) are available. Further, if one extrapolates, say, the main components of GDP from a benchmark year to a later year, one could simply add up these components to obtain an estimate of GDP. This estimate of GDP would not be the same as that using the national growth rate of GDP. The reason for this is that the national growth rates of components are in one case being weighted by the shares of GDP in international prices and in the other by shares at national prices. The case for extrapolating components at international prices to obtain GDP growth is that it most closely replicates what a new benchmark estimate will produce. The case for using the national growth rate of GDP, and perhaps distributing components so as to preserve additivity, is that it does preserve the national growth rate. At present, there is not a recommended practice, and the method used is likely to depend on the specific purpose for which the extrapolation is being carried out.

16/ Meetings were held at EUROSTAT in June 1989 and at OECD in June 1990. These expert group meetings were jointly sponsored by EUROSTAT, OECD and the Statistical Office of the United Nations Secretariat. Reports of the meetings are available from any of the secretariats.

17/ That is, the direct value of (B/A) in the first row is 5.01, while (B/A )A is 10.206 (C/A)/2.021 (C/B) = 5.05; in the second row the direct value is 5.19 and the indirect is 4.31.

18/ In the EKS method, the importance assigned to individual price observations is variable and is not self-evident. Even when the asterisk system is used, the importance assigned to individual prices will depend on the number of observations and on whether they are marked with an asterisk (*). Further, the weight given to indirect price ratios will normally not depend on the number of price observations involved unless explicit weighting is employed. This last problem is also present in the CPD method, where it has been explicitly treated by assigning the same weight to each country so that each price for a country will receive a weight inversely related to the total number of prices for a basic heading for the country. While this could be done in EKS, in applications of EKS it has not been carried out.

19/ Citations to Geary and early phases of the ICP are provided in a volume reviewing aggregation methods (Hill, 1982).

20/ Other systems of weighting are discussed in annex II.

21/ In the phase I-III reports, the results of seven different aggregation methods are reported at the GDP level. These results allow one to gain an impression of how sensitive the results are to the method of aggregation used. See, for example, Kravis, Heston and Summers (1982), pp. 96-97.

22/ If countries within a region retain their relationship at the GDP level obtained from a regional aggregation, then when they are linked to the world comparison some compromise must be adopted. There are several ways to carry out such a linking, two of which are discussed in United Nations, Economic Commission for Europe (1985) and United Nations and EUROSTAT (1986).

23/ Another reason that EC is moving towards annual estimates is because successive benchmark estimates do not necessarily give results that are consistent with the deflated growth estimates of the countries. In effect, by moving to annual estimates, EC will be generating purchasing-power parity estimates that are more consistent with the national deflation practices of the countries.

24/ There are several ways one could do this. One method would be to take the extrapolated value of GDP for a country in a particular year, say Italy in 1990, as a ratio to the value for the United States, the OECD numeraire. Similarly, calculate the same ratio converting Italy's lira GDP in 1990 in current prices at exchange rates relative to current United States GDP. The ratio of the GDPs at exchange rates to the ratio at real 1985 dollars for 1990 gives an estimate of the comparative price level of Italy for 1990, which when multiplied by the exchange rate yields the estimated PPP of Italy for 1990.